How might a digital whiteboard work as a thinking tool?

In short: Interaction with “representations” on a whiteboard canvas can trigger learning processes. This phenomenon has been extensively observed and documented in child development. As it turns out, it exists across age groups and is relevant among adult learners as well. 

In order to understand how cutting-edge collaborative whiteboards support cognition, one only needs to consider childhood and observe how thinking evolves during play. 

Imagine a two-year-old manipulating physical blocks. While playing, the young brain builds up mental constructs of independent objects. These are created stage by stage, integrating elementary action schemes gained from actual experiences within the environment. This is how things like tower-building can happen.

Soon after the assimilated concepts are accommodated in brain structures, it becomes possible for the child to use “abstractions” to think through actions without the need to physically manipulate objects. This is essentially where most adults are — we have a good understanding of how wooden blocks work.

The canvas of a digital whiteboard (a virtual space) makes it easy to represent real objects with pictures or other forms, such as symbols or drawings, while not being constrained by the real environment (a physical space). These are called “first-order representations”.

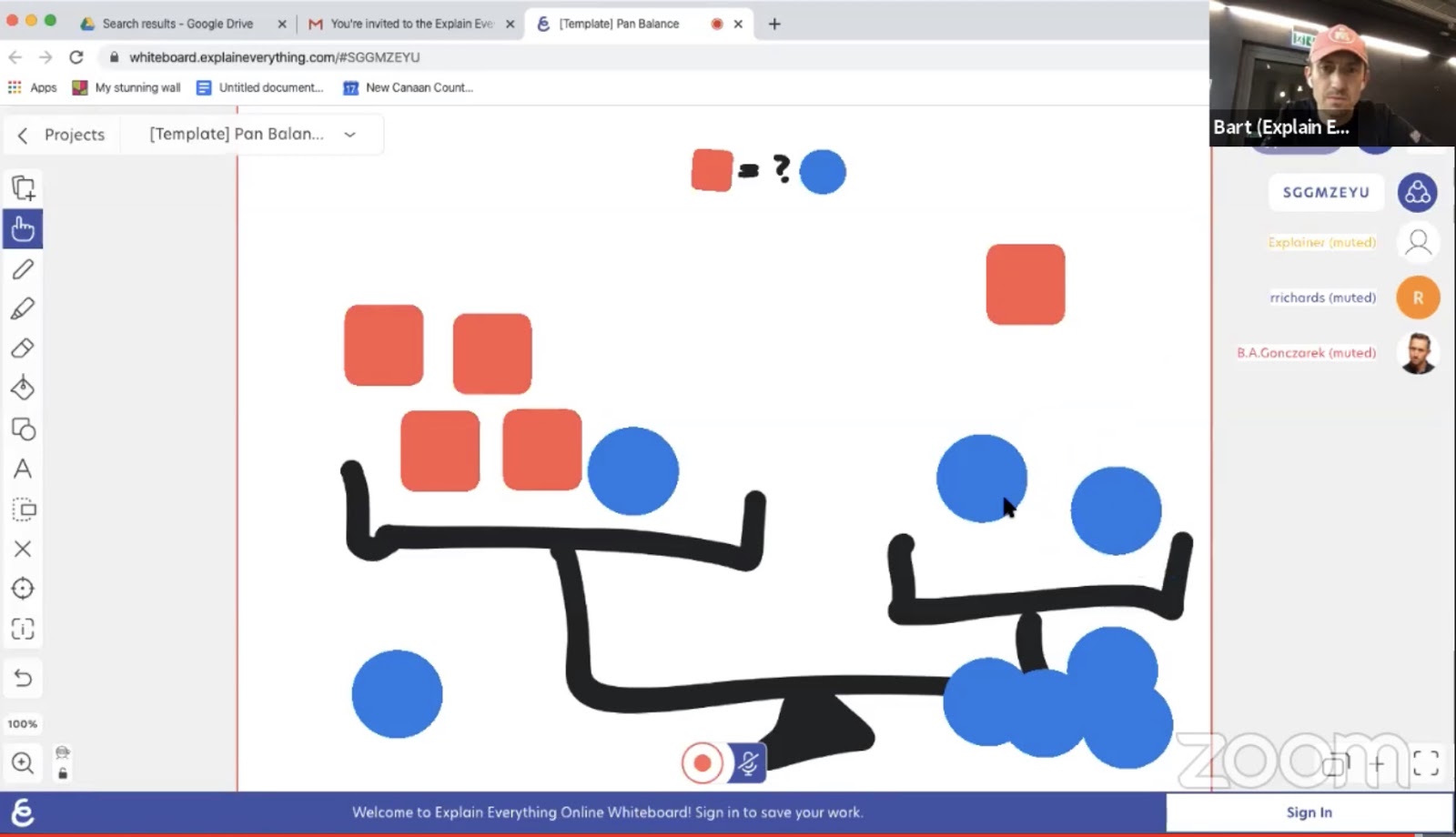
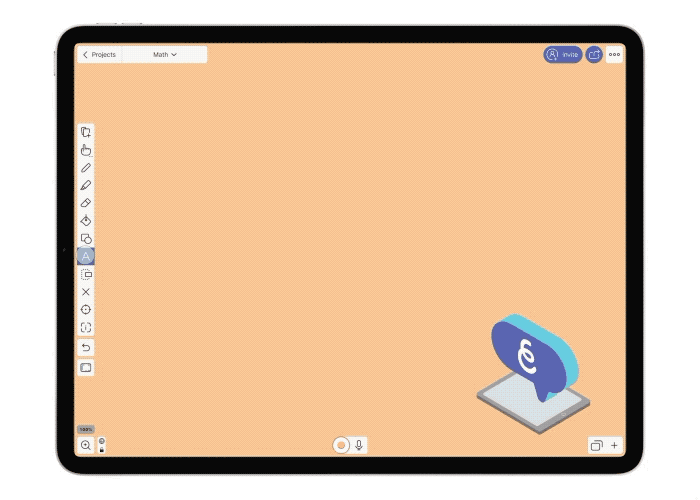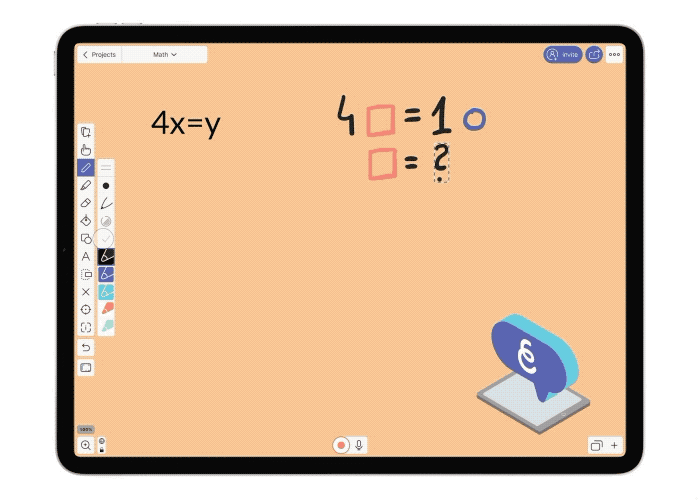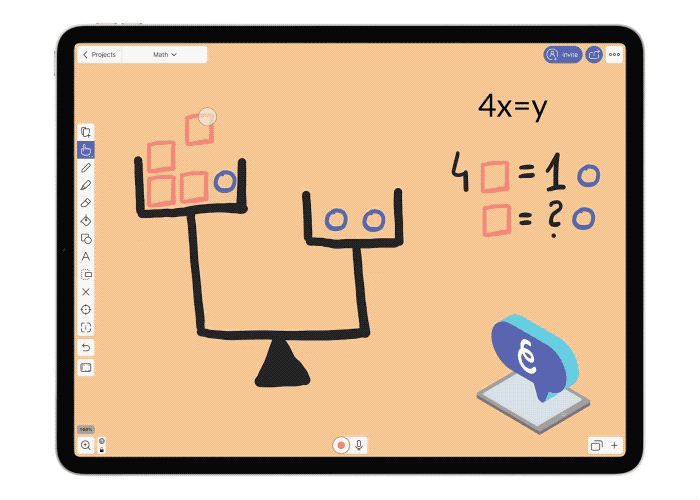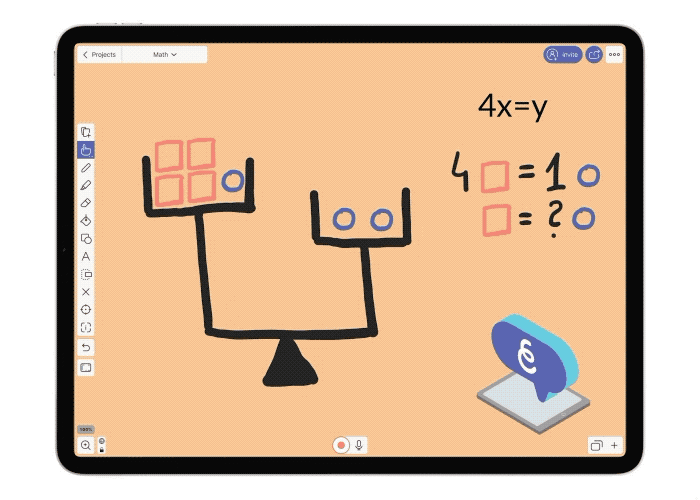
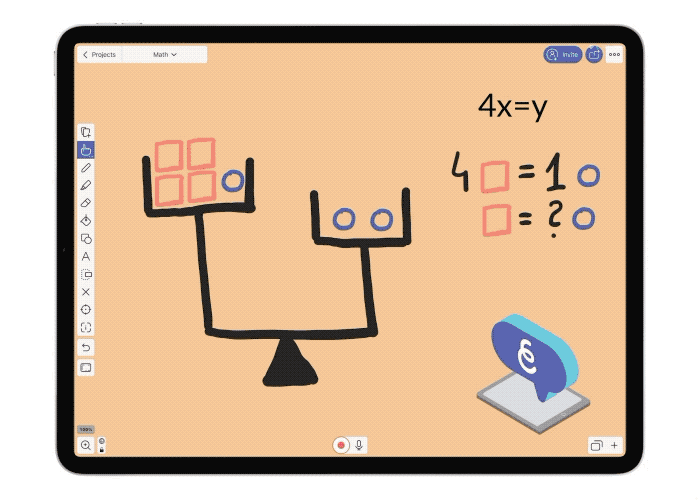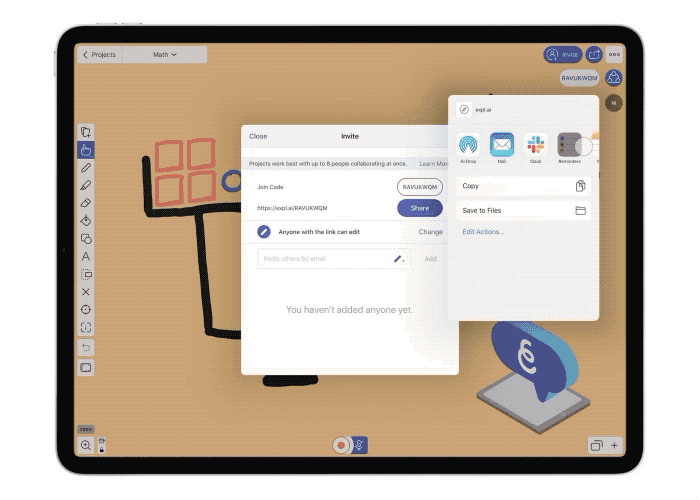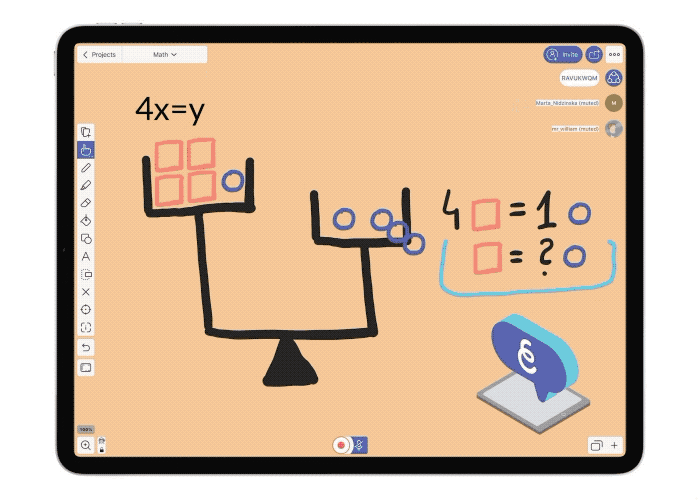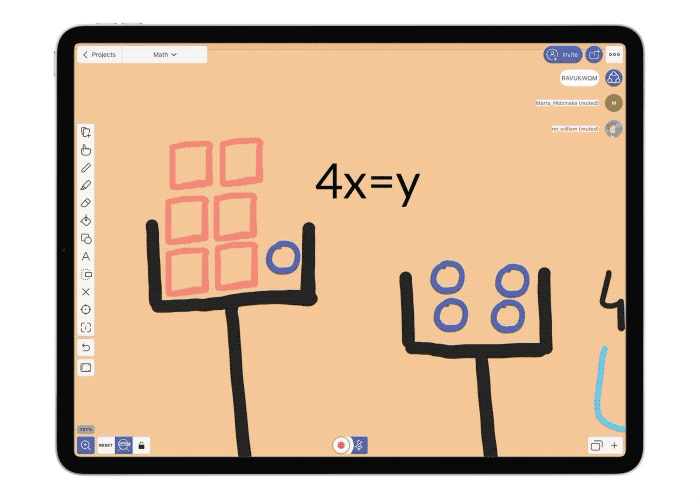
Video: Reshan demonstrates using a pan balance for early algebraic concepts using first-order representations (link to the precise moment of our livestream).

Steps to use first-order representations: 

Step 1: Use the Add tool and select Camera for representing an object with a picture.
Step 2: Take a picture and cut the element you need as a representation from the image. 
Step 3: Use the Inspector tool to duplicate the element if more instances are needed.

Going beyond simple abstractions

Now think of numbering objects using counters or chips, with representing each object. Using counters is a symbolic action that can be traced back at least to the 8th millennium B.C.E. Back then, counters were used to document a “one-to-one” relation between concrete objects and marks on a clay tablet.

Counters can play an important role in cognitive development. These are essentially “second-order representations”, or representations that are indirectly related to objects and actions. They are the cornerstone for the higher-order structures emerging from their usage, for example in algebra, where a set of symbols reflects arithmetical operations.

The digital whiteboard acts as a bridge between direct representation and abstract symbolic systems of knowledge. The canvas allows you to create a direct representation of any items, and, if needed, use them alongside symbolic representations in order to gradually develop understanding — from simple to more abstract and complex ideas.

Example of using a whiteboard for explaining elementary algebra. 

Steps to use higher-order representations: 

Step 1: Use the Text tool or Draw tool to add symbols.
Step 2: Each text or drawing is a separate object that can be manipulated. To draw several independent symbols, use a “tick” to separate them.
Step 3: With the hand tool, you can use each independent object on the canvas. Use the grouping functionality in Inspector to form clusters of related objects. 

Using the digital whiteboard to develop thinking together 

A digital whiteboard is an amazing thinking tool, the ultimate medium to use for the fundamental processes of building understanding across different fields. It is a powerful tool to actively support cognition, one which allows the user to witness transformations of less or more abstract representations through the actions they take. 

Steps to open up a whiteboard in order to explain collaboratively

Step 1: Use the Invite button to open up a whiteboard for access.
Step 2: Share your code so others can connect. The code can also be used as a connection link if you add expl.ai/ at the beginning. 
Step 3: Once connected, you can talk with your viewers in real time and use tools to explain and build audience understanding.

In the end, it’s actions that shape thinking structures, which in turn become more general and applicable to ever richer content. The application of these structures depends on the context; however, the underlying process is similar to what we just illustrated using elementary algebra as our example. 

To read more: follow ideas of Max Wertheimer and Jean Piaget, pioneers of cognitive psychology. Piaget documented the mechanism of “reflective abstractions”,  Wertheimer pioneered “constructivist structuralism” and broke new ground with his  Productive Thinking book as a result of his collaboration with Albert Einstein.